Physical Chemistry English course        .  course homepage

 Midterm Exams and Solutions
1. Write the second postulate of thermodynamics according to Callen.
(6 points)

2. Show that using 'formal relations'. (6 points)

3. Calculate the difference deltaH - deltaU for graphite/diamond transition at 650 K temperature and 500 kbar pressure. Hint: Molar volumes can be calculated from densities. Densitygraphite = 2.27 g cm-3 and densitydiamond = 3.52 g cm-3.
(4 points)

4. Heat capacity of copper changes with temperature according to equation

cp/J K-1 mol-1 = 6.28 10-3T/K + 22.59

Calculate the amount of heat needed to rise the temperature of 1 kg copper from 300 K to 1300 K. Mcu = 63.55 g mol-1.
(4 points)

top of page

Midterm Exam 2  (November 18)
(not yet public)

top of page

 Midterm  Exam 3  (December 8)

1. The standard enthalpy of a certain reaction is +125 kJ mol-1 and the standard reaction Gibbs energy is 22 kJ mol-1 at 1280 K. Estimate the temperature at which the equilibrium constant becomes greater than 1.

2. The equilibrium constant for the reaction N2+O2=2NO is 1.6910-3 at 2300 K. A mixture consisting of 5.0 g of nitrogen and 2.0 g of oxygen in a container of volume 1.0 dm3 is heated to 2300 K and allowed to come to equilibrium. Calculate the mole fraction of NO at equilibrium.

3. Calculate the inlet pressure required to maintain a flow rate of 8.70 cm3 s-1 of nitrogen at 300 K flowing through a pipe of length 10.5 m and diameter 15 mm. The pressure of gas as it leaves the tube is 1.00 bar. The volume of the gas is measured at that pressure.
4. According to Fick's first law of diffusion . Derive Fick's second law.

top of page

 TakeHome Exam  (December 9)
Atkins: Microproject 1.15: Sulfuric acid solutions and lead electrochemistry.

top of page

Optional Exam  (December 22)

1) Mr Sowanso decided to reduce his heating budget, so made installed the best available heat pump, working in an Carnot cycle. He used the nearby river as a cold reservoir.

a) Calculate the efficiency of the heat hump (), when the temperature of the environment (river) is 4C, and room temperature is 24C.

On a cold morning a fuse blew, the heat pump halted and the temperature in the house fell to that of the environment, 4C. When Mr Sowanso finally got home, he replaced the fuse and switched the heating on.

b) What was the efficiency of the heat pump right after the start?

After one hour the temperature rose to 14C.

c) Calculate the average efficiency of the heat pump during this period. (Use the definition given in a)).

d) Calculate the time Mr Sowanso had to wait to reach the usual room temperature (24C), if he left the heat pump on.

Hints: Neglect the heat loss through the walls of the house.

Tips for the integration in c) and d) :

  

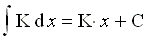
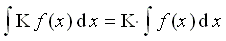
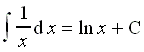
2) Figure 1 shows a device to study surface tension. Initially, the device is filled with water to the level in the left cylinder marked by the lower dotted line, which is the zero point of the vertical scale. (This cylinder has a very large diameter that surface effects on its walls should not be taken into account.) The capillary on the right has a diameter of 1 mm, and it is 10 cm long above zero level.

a) How does the level of the water in the capillary depend on the level in the left-side cylinder if more water is added into the device?

b) How does the shape of the surface (contact angle) of the liquid in the capillary depend on the level in the other side?

Use the following data: g = 9.81 m/s2 rwater = 1000 kg /m3 g water = 72·10-3 N/m

Figure 1 

3) The bismuth-cadmium phase diagram is of interest in metallurgy, and its general form can be estimated from expressions for the depression of freezing point. The metals are mutually insoluble as solids. Calculate the eutectic composition and temperature, using the following data: Tf(Bi) = 544.5 K, Tf(Cd) = 594 K, fusH(Bi) = 10.88 kJ mol-1, fusH(Cd) = 6.07 kJ mol-1.

with .
 
top of page